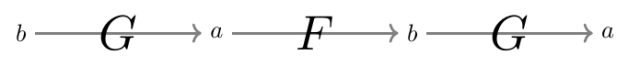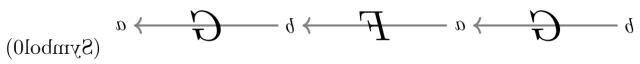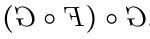Reading Bolzano. Part 1.

The next installment brings few surprises. Bolzano argues for dividing knowledge into parts, and for interlinking those parts, within reason. It is a little surprising (to me) that Bolzano that early in his text defines what he means by ‘Wissenschaft’, and by a rather flexible definition: whatever is deemed worthy to be presented in a book he demands to be permitted to call ‘Wissenschaft’:

 

(This installment brought an innovation in form: the colors correspond to the portions of the text translated. Apart from that, the colors are not intended to mean anything.)

Mathematical Aphorisms. Part 6.

It seems underemphasized that the parsimony of ZFC in the way of ‘types’ (having only one sort called sets) has the following practical and notational virtue: you can get by with a single type of the quantifier , and a single type of the quantifier ; multi-sorted formalisms need multiple sorts for each of and , respectively. Why? Because otherwise the semantics do not work. (You can find a suitable example for yourself.)

Reading Bolzano. Part 0.

This serial will provide, in many and short installments, created every now and then, provide a new translation into English of, and commentary on, the German original of Bernard Bolzano’s 1837 classic book ‘Wissenschaftslehre’.

I omit the front-matter and table of contents, and start with the introduction.

Bolzano begins with a rather conventional (and, I think, even in Bolzano’s time, rather unsurprising) Ὁ βίος βραχύς, ἡ δὲ τέχνη μακρή:

 

 

To be continued.

 

 

 

 

Higher Trees Today. Part 0.

For the time being, the installments of the serial ‘Higher Trees Today’ will focus on three kinds of higher trees:

• Contractible Spaces of Choices (c.s.c.)
• Diestel–Oum decompositions (d.o.d.)
• Kalai trees (k.t.)
• Masbaum-Vaintrob trees (m.v.t.)

Of course, while the latter three are quite unambiguously-defined and attested mathematical definitions, the first is a highly context-dependent notion (that anyone can apply to their favorite context, mathematical or not). Perhaps more precisely put: each of the latter three form a category, or perhaps even more precisely put, each of the latter three are structures in the usual sense of model theory, while the first is not known to either be a category or a class of structures, in any reasonable way. More coming soon.

Vignettes. Part 13.

There probably is no better illustration of the vagaries of notational fashion than the fact that nowadays, the numeral 1 is commonly used to denote the (conceptually very important) terminal category, while George Boole 170 years ago used 1 to denote a universe:

(p. 15 in George Boole, The mathematical analysis of logic : being an essay towards a calculus of deductive reasoning, 1847, Macmillan, Cambridge)

Vignettes. Part 12.

Did you know that mathematics will provide you with more-or-less standardized tools to usefully express commonly-occurring ideas? For exaple, the following illustration contains at least three examples of this: (0) the symbol for boundaries of things, the symbol to symbolize a process leading from a cause to an effect, and, of course, the good old Cartesian coordinate system, a tool usually credited to the publication of La Géométrie over 380 years ago, a tool of expressing ideas without which the following illustration would have taken much longer to construct.

Mathematical Aphorisms. Part 5.

Perhaps the best example¹ of a sound and complete proof system for a general audience are the three Reidemeister moves.

^{1 Calling Reidemeister moves a ‘proof system’ goes back at least to Avi Wigderson’s 2006  ICM contribution.}

Mathematical aphorisms. Part 4.

Bicategories are useful because they allow to constructively compare comparisons.

Vignettes. Part 11.

There is a body of results in higher category theory which this expository blog post will present you a toy version of.

Not to bias this post too much, and not to slight said body of results (they actually are much more substantial than the following story might make you believe), I will not say what technical term said results usually go by. Here I will call them robustness theorems. Disclaimer: this is non-standard terminology.

What I really mean is a term similar to the term Tauberian theorems in number theory: an umbrella term binding together a bundle of similar results.
So again, I won’t tell you what these theorems are usually called—only so much: (0) the analogy with Tauberian theorems is rather apposite (the two bundles of results are spiritually quite similar) in that this is about giving a reasonable meaning to expressions  (1) I here will call them by the unusual term robustness theorems.

So what is the simplest robustness theorem, the toy robustness theorem?
To my mind, arguably, it is the following.

Suppose you know a person P.

Suppose it is understood what the two symbols and mean in the communication between you and P. That is, you know how to interpret both and .

However, the symbols  and are considered nonsense; you will never be satisfied to have made sense of something P sends to you unless you have assembled a well-formed expression involving only and .

Now suppose a person P sends to to you a string of symbols.

Suppose what you are being flashed on your display is

(Symbol0)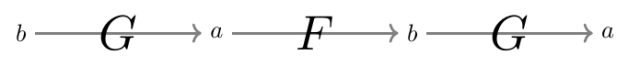

Now what to make of that? This symbols itself is evidently sort-of-a-finite-string
of symbols, quite-string-like indeed. But what is it supposed to mean?
In principle, of course, you are free to interpret in any way you want.
You might interpret it as true, for example.
Yet this is not the intended interpretation.
There is something that this symbol was made for.

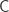It might not surprise you that (Symbol0) is intended to represent one morphism   in a category , with intended to be assembled from the morphisms and .
It will also not surprise you that here, ’assembled from’ does not mean
’assembled in any way you like’, not even ‘in any meaningful way’ .

While logically there is no reason why the above geometric picture must  mean the morphism 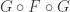 , there are customs regarding how the assembling should work, and those demand the interpretation .

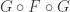In principle, you could define any crazy interpretation of the above symbol, for example would also define a morphism 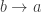there is nothing logically wrong with such a deviant rule, but it does not give the intended interpretation. (It would be similar to having a private rule to interpret the string ‘seventeen’ to mean the number .)
In short, we have to give a rule (for brevity, we will take ‘rule’ to be a convenient monosyllabic synonym for the tetrasyllabic ‘algorithm’) for how to assemble expressions like (Symbol0) into one morphism of a category (to be precise: this, too, is an arbitrary choice-of-semantics that we make here: for the sake of this expository post, we just decide to only interpret expressions in humble 1-categories, while the robustness theorems apply to higher categories), and this rule should be generally applicable and, logically-consistent.

Let us try to make such an interpretation-rule:

(R0) Start at the left of the expression-to-be-interpreted, move rightwards, select the first letter you hit, hold it, interpret it as the name of a morphism in a 1-category , go on moving rightwards until the next,
hold it, interpret it accordingly, compose the two you morphisms you hold via the composition-function of 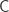to get a single morphism, hold it, go on moving to the right, repeat all of this, until you have reached the right-hand end of the symbol. The morphism-that-is-the-iterated-composite is the interpreation of the expression.

Applied to (Symbol0), (R0) gives us the interpretation .
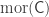Now comes the catch, an alternative scenario: suppose you were being flashed—perhaps due to some technical malfunction, or because you are standing on the other side of a window pane that P is scribbling (Symbol0) on—the symbol

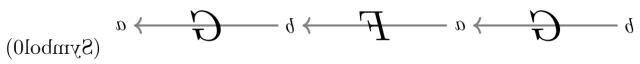
Now what sense to make of that?

Being human, you might recognize this to be just the mirror-image of (Symbol0), and derive your interpretation from this observation, and the above procedure.

Yet, for the sake of argument, suppose you are being not very perceptive today, and perhaps currently so much in awe of the idea of non-text syntax that you cannot do better than to timidly and rigidly stick to your interpretationi rule (R0) when interpreting .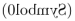

You are then led to interpret 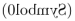to the composite

(TemporaryInterpretation0)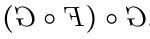

 

 

which, however, does not yet make sense under the hypotheses stipulated in the beginning (namely that the symbols 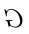and in an interpretation of P’s messages are not acceptable to you).

Then there are the two following reasonable interpretation-options open to you, to give acceptable meaning to (TemporaryInterpretation0): either you reflect each variable in 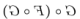separately to arrive at , which happens to be the same as what you interpreted (Symbol0) to.
However, it is also reasonable, and arguably more reasonable, that you simply reflect the expression 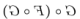 from (TemporaryInterpretation0) in its entirety about the vertical axis to arrive at .
So, in summary, in two scenarios you ended up with the interpretation , while in another scenario (in a sense, in one-out-of-three possible scenarios for the communication process), you ended up with .
Fortunately, the usual axioms of 1-category theory contain an equality statement
expressing associativity of composition, telling you that, in particular, .
So if you interpret (Symbol0) in any one of the above ways,

• the axioms of 1-categories are robust to some of the vagaries of communication, provided that customary interpretation rules are used,

in that

• the resulting morphisms came out the same, despite your rigid rule (R0),
  and despite the transmission error that handed you a mirror image, and despite the arbitrary choice of what to make of reflected letters.

We hasten to add that, needless to say

• no non-trivial axiomatic system can be robust against all possible non-customary and possibly crazy interpretation rules (like, say, a rule which would interpret the mirror-image to mean ).

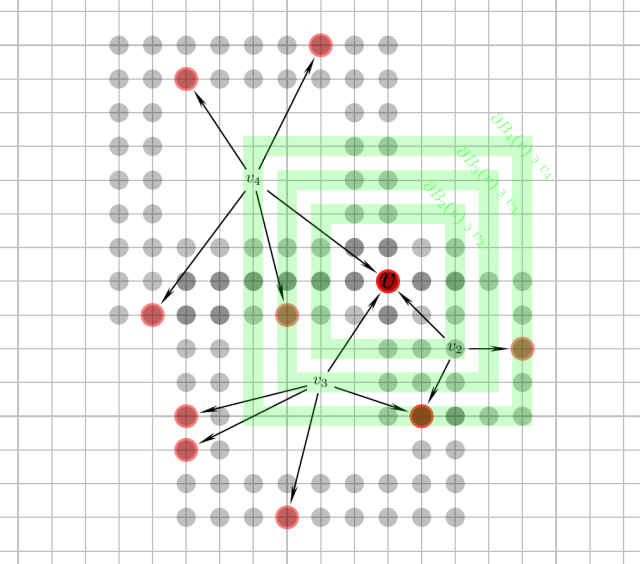
One can illustrate this above story in an informal ‘diagram’ (disclaimer: this
diagram is not known to be a mathematical object; the arrows have different sorts; not all arrows in the following are claimed to be morphisms of a category):

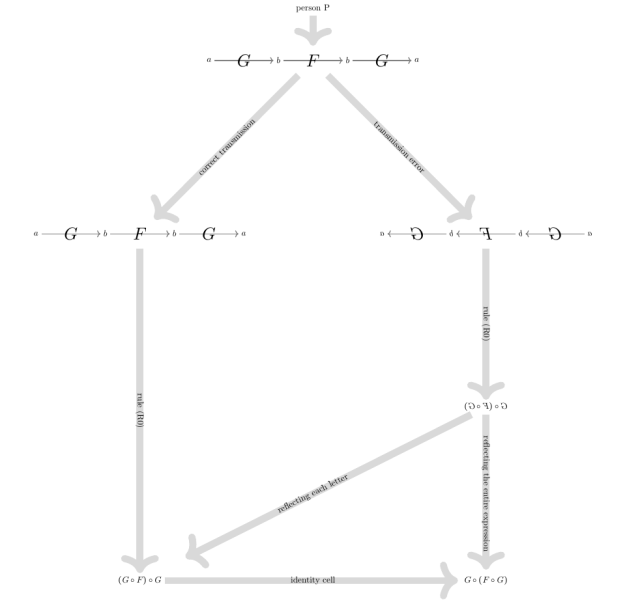
Here, ‘identity cell’ is a technical term, more or less synonymous with ‘equality’. (In case you are interested, ‘equality’ rather means something like a logical judgement, with which one just declares two things to be equal, while ‘identity cell’ means something like evidence, emphasizing that there is some specified amount of data in a standardized form, witnessing that the two things in question are equal).

To sum up, we have our toy robustness theorem:

• the axioms of 1-category theory make the transmission of the one-member class of symbols (Symbol0) robust against (0) rather arbitrary distortions of the symbol by Euclidean plane isometries, and quite some more distortions, and (1) a rigid interpretation rule like (R0). The resulting morphism of the category is exactly the same.

 

Mathematical Aphorisms. Part 3.

With the discovery of higher dimensional algebra, the standard technical term linear equation accidentally has acquired a non-standard second meaning. In particular:

old-sense linear equationnew-sense linear equationany old-sense equation.